

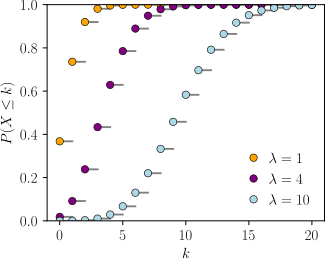
For example, if the average number of people who buy cheeseburgers from a fast-food chain on a Friday night at a single restaurant location is 200, a Poisson distribution can answer questions such as, "What is the probability that more than 300 people will buy burgers?" The application of the Poisson distribution thereby enables managers to introduce optimal scheduling systems that would not work with, say, a normal distribution. Tables of the Poisson Cumulative Distribution. x: A vector of elements whose cumulative probabilities you would like to determine given the distribution d. This calculator will compute the cumulative distribution function (CDF) for the Poisson distribution, given the number of event occurrences and the expected. Many economic and financial data appear as count variables, such as how many times a person becomes unemployed in a given year, thus lending themselves to analysis with a Poisson distribution.Ī Poisson distribution can be used to estimate how likely it is that something will happen "X" number of times. d: A Poisson object created by a call to Poisson().is the shape parameter which indicates the average number of events in the given time interval. Poisson distributions are used when the variable of interest is a discrete count variable. Statistics - Cumulative Poisson Distribution.Recall that the mathematical constant e is the. Tables of the Poisson Cumulative Distribution The table below gives the probability of that a Poisson random variable X with mean is less than or equal to x. If X is a Poisson random variable, then the probability mass function is: f ( x) e x x for x 0, 1, 2, and > 0, where will be shown later to be both the mean and the variance of X. The following is the plot of the Poisson cumulative distribution function with the same values. Let X equal the number of students arriving during office hours. A Poisson distribution, named after French mathematician Siméon Denis Poisson, can be used to estimate how many times an event is likely to occur within "X" periods of time. The formula for the Poisson probability mass function is.Wolfram Knowledgebase Curated computable knowledge powering Wolfram|Alpha. Evaluates the cumulative distribution function for a Poisson distribution with mean parameter lambda. Exam- ple: to find the probability P(X 3) where X has a Poisson. Wolfram Universal Deployment System Instant deployment across cloud, desktop, mobile, and more. Table shows cumulative probability functions of Poisson Distribution with various. Duality of events between waiting times and stochastic process. The Poisson distribution with parameter is a discrete probability distribution, with positive values and whose probability mass function is given by f(k,). Wolfram Data Framework Semantic framework for real-world data. Cumulative Distribution function of a poisson distribution in terms of it's parameter.


 0 kommentar(er)
0 kommentar(er)
